Intonation VI

| Previous article | Next article |

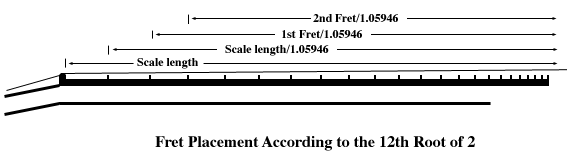
I got a call from one of my readers that made it clear to me that I'd screwed up: I had spent so much time explaining the details, I hadn't got the big point across. This has to be the worst sin a technical writer can commit. So for those of you who have understood everything so far, I apologize for beating a dead horse. But for those of you who were left scratching your heads after 5 articles of reading, I'd like to resurrect this poor steed and give it one more good whack. Intonation 0.5 It's not that I completely left out the crux of my argument, I just didn't give it enough emphasis. So, quoting from Intonation IV, here it is: "... the act of fretting the string bends its pitch sharp, to a degree determined by the gauge and material of the string, action height, scale length and open-string pitch." I'd like to fine-tune that explanation just a bit by adding the following: The relative intonation of any note on the guitar is determined by the position of that fret relative to the nut and saddle, and how much that string is bent sharp by fretting that note. It's a problem in two dimensions. Horizontally, the relative placement of the nut, frets and saddle lay out the equal-tempered scale. Vertically, the distance from each string to each fret determines how much the string goes sharp when fretted. New Horizons As I explained in Intonation IV, fret placement is calculated mathematically according to a division of the octave into 12 logarithmically equal parts. The scale length is first established by the distance from the nut to the saddle. Then that scale length is divided by the 12th root of 2 (1.05946...) to locate the first fret. Each successive fret is located by dividing the remainder of the scale length by that number. When you get to the 12th fret, you have divided by the 12th root of two, 12 times, which is to say you've divided by two. Halving the length doubles the pitch, giving you a perfect octave at the 12th fret. The fretboard is slotted for the frets according to this formula, and most modern luthiers and guitar factories cut the slots to a very high degree of accuracy, so let's assume that the frets on most any fretboard are correctly placed. But the fret slot positions are calculated relative to the nut, so all of this careful fret placement is for nought if the nut is incorrectly placed. Ignoring the factor of strings bending sharp when fretted, let's look at an extreme case of nut misplacement. Let's suppose that I were so careless as to cut 3/4" off the nut end of the fretboard. Now the nut is actually about halfway between its correct position and the first fret. I've drawn a little mark on the string to show where the fret should contact it relative to a correctly placed nut: If I were then to intonate this guitar by the traditional method of comparing the harmonic at the 12th fret with the fretted pitch there, I might think everything was be fine. But the fact is, every note below the 12th fret will be flat, and every note above the 12th fret will be sharp! This is an extreme example, but misplacing the nut by a few thousandths of an inch can audibly throw off intonation. So nut placement is critical to the intonation of every note on the neck, not just the open strings. But what is correct nut placement? As I explained in Intonation IV, the nut placement must be compensated because the open strings are the only notes on the neck that are not fretted, and therefore are not bent sharp. This is why the nut must actually be placed slightly closer to the saddle than the mathematical ideal. In the next article... In Intonation VII I'll revisit the effect on intonation of strings bending sharp when fretted. Meanwhile, I'll look for your suggestions and questions in my mailbox... E-Mail Me And for Pete's sake, somebody out there tell me when I'm not making sense!.

| Previous article | Next article |